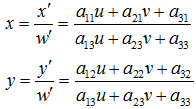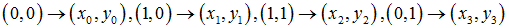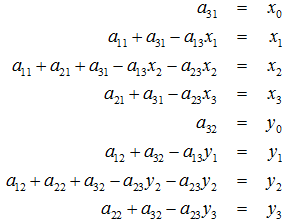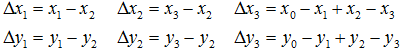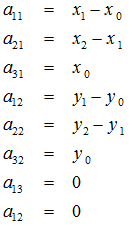图像处理中的投影变换（Perspective Transformation）

大家好，欢迎来到IT知识分享网。

透视变换(Perspective Transformation)是将图片投影到一个新的视平面(Viewing Plane)，也称作投影映射(Projective Mapping)。通用的变换公式为：

u,v是原始图片左边，对应得到变换后的图片坐标x,y,其中。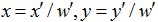
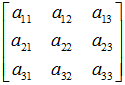
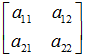
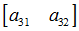
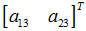
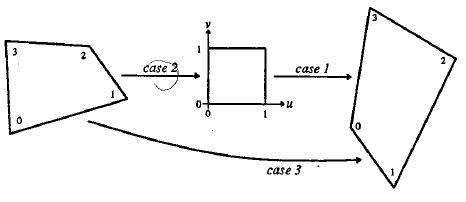
变换矩阵可以拆成4部分，表示线性变换，比如scaling，shearing和ratotion。用于平移，产生透视变换。所以可以理解成仿射等是透视变换的特殊形式。经过透视变换之后的图片通常不是平行四边形（除非映射视平面和原来平面平行的情况）。

所以，已知变换对应的几个点就可以求取变换公式。反之，特定的变换公式也能新的变换后的图片。简单的看一个正方形到四边形的变换：
变换的4组对应点变换可以表示成：

根据变换公式得到：

都为0时变换平面与原来是平行的，可以得到：

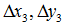
（将上面最后一行中的 a12 = 0 修改为 a23 = 0）

不为0时，得到：

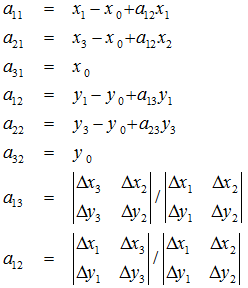
（将上面最后一行中的 a12 = …… 修改为 a23 = ……）

代码：

PerspectiveTransform::PerspectiveTransform(float inA11, float inA21, 
                                           float inA31, float inA12, 
                                           float inA22, float inA32, 
                                           float inA13, float inA23, 
                                           float inA33) : 
  a11(inA11), a12(inA12), a13(inA13), a21(inA21), a22(inA22), a23(inA23),
  a31(inA31), a32(inA32), a33(inA33) {}

PerspectiveTransform PerspectiveTransform::quadrilateralToQuadrilateral(float x0, float y0, float x1, float y1,
    float x2, float y2, float x3, float y3, float x0p, float y0p, float x1p, float y1p, float x2p, float y2p,
    float x3p, float y3p) {

  PerspectiveTransform qToS = PerspectiveTransform::quadrilateralToSquare(x0, y0, x1, y1, x2, y2, x3, y3);
  PerspectiveTransform sToQ =
    PerspectiveTransform::squareToQuadrilateral(x0p, y0p, x1p, y1p, x2p, y2p, x3p, y3p);
  return sToQ.times(qToS);
}

PerspectiveTransform PerspectiveTransform::squareToQuadrilateral(float x0, float y0, float x1, float y1, float x2,
    float y2, float x3, float y3) {

  float dx3 = x0 – x1 + x2 – x3;
  float dy3 = y0 – y1 + y2 – y3;
  if (dx3 == 0.0f && dy3 == 0.0f) {

    PerspectiveTransform result(PerspectiveTransform(x1 – x0, x2 – x1, x0, y1 – y0, y2 – y1, y0, 0.0f,
                                     0.0f, 1.0f));
    return result;
  } else {

    float dx1 = x1 – x2;
    float dx2 = x3 – x2;
    float dy1 = y1 – y2;
    float dy2 = y3 – y2;
    float denominator = dx1 * dy2 – dx2 * dy1;
    float a13 = (dx3 * dy2 – dx2 * dy3) / denominator;
    float a23 = (dx1 * dy3 – dx3 * dy1) / denominator;
    PerspectiveTransform result(PerspectiveTransform(x1 – x0 + a13 * x1, x3 – x0 + a23 * x3, x0, y1 – y0
                                     + a13 * y1, y3 – y0 + a23 * y3, y0, a13, a23, 1.0f));
    return result;
  }
}

PerspectiveTransform PerspectiveTransform::quadrilateralToSquare(float x0, float y0, float x1, float y1, float x2,
    float y2, float x3, float y3) {

  // Here, the adjoint serves as the inverse:
  return squareToQuadrilateral(x0, y0, x1, y1, x2, y2, x3, y3).buildAdjoint();
}

PerspectiveTransform PerspectiveTransform::buildAdjoint() {

  // Adjoint is the transpose of the cofactor matrix:
  PerspectiveTransform result(PerspectiveTransform(a22 * a33 – a23 * a32, a23 * a31 – a21 * a33, a21 * a32
                                   – a22 * a31, a13 * a32 – a12 * a33, a11 * a33 – a13 * a31, a12 * a31 – a11 * a32, a12 * a23 – a13 * a22,
                                   a13 * a21 – a11 * a23, a11 * a22 – a12 * a21));
  return result;
}

PerspectiveTransform PerspectiveTransform::times(PerspectiveTransform other) {

  PerspectiveTransform result(PerspectiveTransform(a11 * other.a11 + a21 * other.a12 + a31 * other.a13,
                                   a11 * other.a21 + a21 * other.a22 + a31 * other.a23, a11 * other.a31 + a21 * other.a32 + a31
                                   * other.a33, a12 * other.a11 + a22 * other.a12 + a32 * other.a13, a12 * other.a21 + a22
                                   * other.a22 + a32 * other.a23, a12 * other.a31 + a22 * other.a32 + a32 * other.a33, a13
                                   * other.a11 + a23 * other.a12 + a33 * other.a13, a13 * other.a21 + a23 * other.a22 + a33
                                   * other.a23, a13 * other.a31 + a23 * other.a32 + a33 * other.a33));
  return result;
}

void PerspectiveTransform::transformPoints(vector<float> &points) {

  int max = points.size();
  for (int i = 0; i < max; i += 2) {

    float x = points[i];
    float y = points[i + 1];
    float denominator = a13 * x + a23 * y + a33;
    points[i] = (a11 * x + a21 * y + a31) / denominator;
    points[i + 1] = (a12 * x + a22 * y + a32) / denominator;
  }
}